Advanced Materials for Magnetic Cooling
Prof. V. K. Pecharsky, Prof. K. A. Gschneidner, Jr.
Ames Laboratory, Iowa State University
Material Matters 2007, 2.4, 4.
Introduction
Today, near-room-temperature refrigeration is almost entirely based on a vapor-compression refrigeration cycle. Over the years, all parts of a commercial refrigerator, such as the compressor, heat exchangers, refrigerant, and packaging, have been improved considerably due to the extensive research and development efforts carried out by academia and industry. However, the achieved and anticipated improvements in conventional refrigeration technology are incremental since this technology is already near its fundamental limit of energy efficiency. Furthermore, chlorofluorocarbons, hydrofluorocarbons, and other chemicals used as refrigerants eventually escape into the environment promoting ozone layer depletion and global warming. In general, liquid chemical-based refrigeration is a major factor contributing to deleterious, cumulative changes in the global climate.
Refrigeration is based on the use of a working body that changes its temperature in response to certain thermodynamic triggers to cool an object. These variations must be achieved quickly, repeatedly, reversibly, and with minimum energy losses. Since a magnetic field effectively couples to magnetic moments of individual atoms in a solid, magnetic field is one of the common thermodynamic variables that can alter the temperature of a magnetic solid. Heating and cooling of soft ferromagnetic materials in response to increasing and decreasing magnetic fields, respectively, has been known since the latter part of the 19th century when Warburg reported small but measurable reversible temperature changes in pure iron in response to magnetic field changes.1 Today, this phenomenon is recognized as the Magnetocaloric Effect (MCE) and materials exhibiting large, reversible temperature changes in response to changing magnetic fields are usually referred to as magnetocaloric materials. The efficiency gain when replacing a mechanical process (compression and expansion of a vapor) with an electronic process (magnetization and demagnetization of a solid) to obtain a reversible change of temperature is substantial, thus making magnetic refrigeration one of the few viable, energy-efficient solid-state cooling technologies.
Magnetocaloric Effect
The magnetocaloric effect occurs when a magnetic sublattice is coupled with an external magnetic field, affecting the magnetic part of the total entropy of a solid. Similar to the isothermal compression of a gas, during which the positional disorder and the corresponding component of the total entropy of the gaseous system are suppressed, exposing a paramagnet near absolute zero temperature or a ferromagnet near its Curie temperature, TC, to a change in magnetic field (B) from zero to any non-zero value, or in general, from any initial value Bi to a final higher value Bf (DB = Bf – Bi > 0) greatly reduces disorder of a spin system. Thus, the magnetic part (SM) of the total entropy (S) is substantially lowered. In a reversible process, which resembles the expansion of the gas at constant temperature, isothermal demagnetization (DB < 0) restores the zero field magnetic entropy of a system. The magnetocaloric effect, therefore, can be quantified as an extensive thermodynamic quantity, which is the isothermal magnetic entropy change, DSM.
When a gas is compressed adiabatically, its total entropy remains constant whereas, velocities of the constituent molecules, and therefore, the temperature of the gas both increase. Likewise, the sum of the lattice and electronic entropies of a solid must change by -DSM as a result of adiabatic magnetization (or demagnetization) of the material, thus leading to an increase (decrease) of the vibrational entropy of the lattice. This brings about an adiabatic temperature change, DTad, which is an intensive thermodynamic quantity that is also used to measure and quantify the magnetocaloric effect.
The Standard Magnetocaloric Material—Gd
For near-room-temperature applications, the rare earth metal Gd is the benchmark magnetic refrigerant material. It exhibits excellent magnetocaloric properties that are difficult to improve upon. Not surprisingly, the metal has been employed in early demonstrations of near-ambient cooling by the magnetocaloric effect.2–4 Gadolinium (263087, 261114, 263060, 691771) was used as the refrigerant powering the first successful proof-of-principle refrigerator device.4 Metallic gadolinium has constituted the whole or at least a major part of every magnetic regenerator bed in nearly every near-room-temperature magnetic cooling machine built and tested to date.5,6
The isothermal magnetic entropy change in Gd, calculated from heat capacity and magnetization data, is shown in Figure 1.7 The MCE computed from the two different types of experimental data match well (as shown by the results for the 2T and 5T magnetic field changes), provided experimental measurements have been performed with sufficient accuracy. Furthermore, Figure 1 shows that as the magnetic field increases, the derivative of the MCE with respect to the magnetic field decreases (both DTad and DSM are nearly proportional to B2/3, i.e. d(MCE)/dB ∝ B–1/3). In other words, the highest specific magnetocaloric effect (i.e. the MCE per unit field change) always occurs near zero magnetic field. The intensive MCE of Gd is illustrated for four different magnetic field changes in Figure 2.7 Similar to DSM, DTad, peaks at TC and d(DTad)/dB is also substantially reduced as B increases. The nearly B2/3 dependence of the DTad of Gd is illustrated in Figure 3, where experimental measurements reported by numerous authors exhibit an excellent fit of the MCE data to the B0.7 behavior.
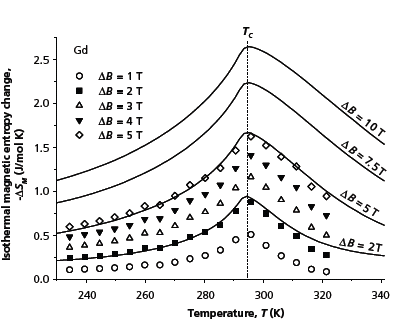
Figure 1.A comparison of the magnetocaloric effect (isothermal magnetic entropy change, DSM) for Gd calculated from magnetization (symbols) and heat capacity data (solid lines).
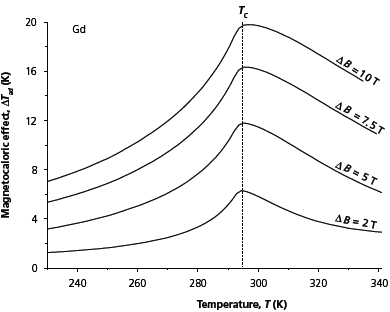
Figure 2.The magnetocaloric effect (adiabatic temperature change, DTad) for Gd calculated from heat capacity data.

Figure 3.The magnetocaloric effect for Gd at its Curie temperature, shown as a function of the final magnetic field, Bf, for Bi = 0. The symbols represent values either measured directly or calculated from heat capacity data by different authors.7 The line is the least squares fit assuming powerlaw dependence of the MCE on the magnetic field.
The behavior of the magnetocaloric effect of Gd illustrated in Figures 1–3 is quite universal for materials with secondorder paramagnetic-ferromagnetic phase transformations. The differences between the MCE in Gd and in those of other second-order phase transition materials mainly lie in differences in the absolute values of the magnetocaloric effect for the same magnetic field change, the temperature of the peak, and how quickly the derivative, d(MCE)/dB, is suppressed by the increasing magnetic field. To illustrate this universality, Figure 4 shows the adiabatic temperature change of a few different magnetocaloric materials, all of which order magnetically via second-order transformations at various temperatures ranging from ~14 K to ~294 K. One of the five materials—elemental dysprosium—orders antiferromagnetically but magnetic fields exceeding ~2T transform the metal into a collinear ferromagnet, thus the behavior of the MCE near the Neél temperature of Dy (261076, 263028, 263036) is nearly identical to that of other ferromagnets.
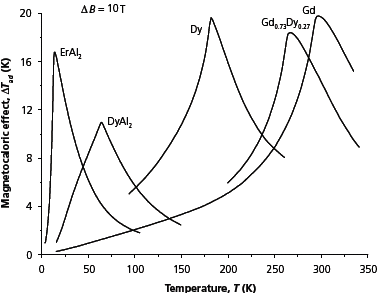
Figure 4.The magnetocaloric effect in ErAl2,8 DyAl2,8 Dy,9 Gd0.73Dy0.27,10 and Gd7 calculated from heat capacities measured in a zero and 10T magnetic field.
Giant Magnetocaloric Effect
Rising interest in both the fundamental science and potential applications of advanced magnetocaloric materials has been sparked by recent discoveries of new compounds exhibiting a magnetocaloric effect much larger (in some cases by a factor of two to three) than those found in previously known compounds, including elemental Gd. The most notable examples that constitute a pool of advanced magnetocaloric materials are FeRh,11 La0.8Ca0.2MnO3,12and Gd5Si2Ge2 and the related Gd5(SixGe4–x) alloys;13,14 the latter references also coined the phrase “the giant magnetocaloric effect” (GMCE) materials. A few years later, several other families of materials have been shown to also exhibit the giant magnetocaloric effect at temperatures close to ambient. These include Tb5Si2Ge2,15 MnAs and MnAs1–xSbx compounds,16 La(Fe1–xSix)13 alloys and their hydrides La(Fe1–xSix)13Hy,17 MnFeP0.45As0.55 and related MnFePxAs1–x alloys,18 and Ni2±xMn1±xGa ferromagnetic shape memory alloys.19
Today, it has been well established that the giant magnetocaloric effect arises from magnetic field induced magnetostructural first-order transformations. Upon the application of a magnetic field, the magnetic state of a compound changes from a paramagnet or an antiferromagnet to a nearly collinear ferromagnet simultaneously with either a martensitic-like structural distortion, or is accompanied by a phase volume discontinuity but without a clear crystallographic modification. When the system undergoes a first-order phase transition, the behavior of the total entropy as a function of temperature reflects a discontinuous (in reality, almost always continuous, except for some ultra-pure lanthanides) change of entropy at a critical temperature, Tc.
The behavior of both the extensive and intensive measures of the giant magnetocaloric effect in first-order phase transition materials is different when compared to the conventional magnetocaloric effect in second-order phase transition compounds, as can be easily seen in Figure 5 when compared to Figures 1 and 2. First, especially for small magnetic fields, the giant magnetocaloric effect is much larger than the conventional MCE (see a recent review by Gschneidner et al.20). Second, the width of the GMCE becomes broader as DB increases, but it broadens asymmetrically on the high temperature side of the magnetic ordering phase transition. Third, as DB increases, both the DSM and DTad increase rapidly for small fields with the corresponding derivatives (∂DSm/∂DB and ∂DTad/∂DB) exhibiting a clear tendency towards saturation. As a matter of fact, when the magnetic field is strong enough to complete the transformation, the magnitudes of the DSM discontinuities remain identical. These discontinuities correspond to the entropies of phase transformations (that include both the magnetic and structural contributions, Figure 5), and the observed modest rise of the background under DSM peaks is due to magnetic field effects on the magnetic entropy of the material in the ferromagnetic state, just as in other materials exhibiting conventional MCE. As was shown recently,9 the calculated magnetic entropy change in Dy in the vicinity of its first-order magnetic phase transition at T = 90 K matches the entropy change of the spontaneous FM → AFM phase transformation measured directly in a zero magnetic field to within 2%.
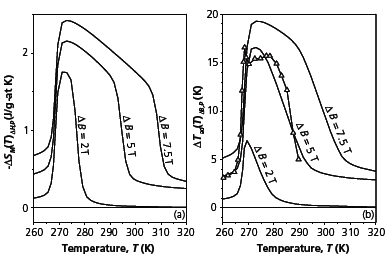
Figure 5.The giant magnetocaloric effects in Gd5Si2Ge2 as represented by (a) extensive (DSM) and (b) intensive (DTad) measures, shown as functions of temperature for three different magnetic field changes: from 0 to 2T, from 0 to 5T, and from 0 to 7.5T calculated from heat capacity data. The open triangles in (b) represent the GMCE measured directly for a magnetic field change from 0 to 5T.
Obviously, the conventional (Figures 1 and 2) and giant (Figure 5) MCE’s are considerably different, and the difference between them should be primarily ascribed to the absence and the presence of a structural change in second-order and first-order materials, respectively. The giant MCE is achieved due to the concomitant change of the entropy during the structural transformation, DSstr. As a result, the observed giant magnetocaloric effect DSM is the sum of the conventional magnetic entropy-driven process (DSm) and the difference in the entropies of the two crystallographic modifications (DSstr) of a solid. In other words, it is the second term of the right hand side of the following equation that is at the core of the giant magnetocaloric effect.
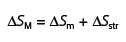
Advanced Magnetocaloric Materials and Other Possible Applications
The discovery of the giant magnetocaloric effect and extensive characterization of multiple families of GMCE materials are indeed extremely important developments both in the science of the magnetocaloric effect and, potentially, in its application to near-room-temperature cooling. The overlapping contribution from the crystallographic and related electronic changes in the lattice may account for 50% or more of the total MCE (as quantified by the isothermal magnetic entropy change) in magnetic fields of 5T and below. More significantly, the relative contribution from the structural entropy change DSstr to DSM increases as the magnetic field decreases so long as the final magnetic field (Bf) is strong enough to complete the magnetostructural transition. We refer the interested reader to the latest review20 for a chart schematically comparing the magnetocaloric effects in first-order phase transition compounds (GMCE materials) and second-order phase transition compounds (MCE materials) and for a list of references (including earlier reviews) where one can find a comprehensive summary describing today’s state-of-the-art magnetocaloric materials.
Bonding, structural, electronic, and magnetic changes, which occur in the giant magnetocaloric effect systems, bring about some extreme changes of the materials’ behavior resulting in a rich variety of unusually powerful magneto-responsive properties in addition to the GMCE. In particular, these include the colossal magnetostriction (which can be as much as ten times larger than that in Terfenol-D), and the giant magnetoresistance (which is comparable to that found in artificial multi-layered thin films). We note here that the giant magnetoresistance observed near the corresponding phase transformation temperatures may be either positive or negative depending upon the nature of the giant magnetocaloric effect material. An easy manipulation of the chemical composition, for example the Si to Ge ratio in Gd5(SixGe4–x) alloys, enables one to precisely tune these materials to display the largest required response almost at any temperature between ~4 and ~300 K. Similar effects may be found when the hydrogen concentration in La(Fe1–xSix)13Hy alloys, or the As to Sb ratio in MnAs1–xSbx compounds is changed.
Advanced magnetocaloric materials, no doubt, should exist in other solid systems where structural changes are coupled with ferromagnetic ordering, and therefore, can be triggered by a magnetic field. Materials designed to maximize the entropy difference between the low-magnetic-field and highmagnetic- field phases, i.e. those that exhibit large entropy of a structural transformation, DSstr, in addition to a large magnetic entropy change DSm, are expected to exhibit the strongest MCE’s in the weakest magnetic fields. Furthermore, it is important that these materials also have large DTad, which can be achieved by maximizing the effect of a magnetic field on the phase transition temperature. Despite numerous studies of first-order phase transition materials, much remains to be learned about the fundamentals of the giant magnetocaloric effect. The most critical issues are how to control both the magnetic and lattice contributions to the phenomenon in order to maximize the magnetocaloric effect in reasonably small magnetic fields (on the order of 1 to 2 Tesla), and how to reduce some potentially deleterious effects such as time dependence and irreversibility associated with the GMCE, thus paving the way to the applicability of these advanced magnetic materials in emerging magnetic refrigeration technology.
Acknowledgments
Work at the Ames Laboratory is supported by the Office of Basic Energy Sciences, Materials Sciences Division of the U. S. Department of Energy under Contract No. DE-AC02-07CH11358 with Iowa State University of Science and Technology.
| Product No. | Product Name | Description | Pricing |
|---|---|---|---|
| LIG2 | QuickLink™ DNA Ligation Kit | joins blunt-end and sticky-end DNA fragments |
References
To continue reading please sign in or create an account.
Don't Have An Account?